
Outside the door, on a line perpendicular to the opening in the door, a jet engine makes a 600-Hz sound. An aircraft maintenance technician walks past a tall hangar door that acts like a single slit for sound entering the hangar. At what angles to the incident direction are the boats inside the harbor most protected against wave action?Ģ9. Ocean waves of 20.0-m wavelength approach the opening straight on. A water break at the entrance to a harbor consists of a rock barrier with a 50.0-m-wide opening. If the separation between the first and the second minima of a single-slit diffraction pattern is 6.0 mm, what is the distance between the screen and the slit? The light wavelength is 500 nm and the slit width is 0.16 mm.Ģ8. (d) Use your answers to illustrate how the angular width of the central maximum is about twice the angular width of the next maximum (which is the angle between the first and second minima).Ģ7. (b) What is the angle of the third-order minimum? (a) If a single slit produces a first minimum at \(\displaystyle 14.5°\), at what angle is the second-order minimum? (b) What is its minimum width if it produces 50 minima?Ģ6. (a) What is the minimum width of a single slit (in multiples of \(\displaystyle λ\)) that will produce a first minimum for a wavelength \(\displaystyle λ\)? (c) Discuss the ease or difficulty of measuring such a distance.Ģ5. (b) What is the distance between these minima if the diffraction pattern falls on a screen 1.00 m from the slit? (a) Find the angle between the first minima for the two sodium vapor lines, which have wavelengths of 589.1 and 589.6 nm, when they fall upon a single slit of width \(\displaystyle 2.00μm\). How far from the center of the pattern are the centers of the first and second dark fringes?Ģ4. Consider a single-slit diffraction pattern for \(\displaystyle λ=589nm\), projected on a screen that is 1.00 m from a slit of width 0.25 mm. (b) What is the highest-order minimum produced?Ģ3. At what angle does it produces its second minimum?
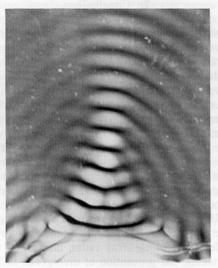
(a) Sodium vapor light averaging 589 nm in wavelength falls on a single slit of width \(\displaystyle 7.50μm\). Find the wavelength of light that has its third minimum at an angle of \(\displaystyle 48.6°\) when it falls on a single slit of width \(\displaystyle 3.00μm\).Ģ2. (b) Find the wavelength of light that has its first minimum at \(\displaystyle 62.0°\).Ģ1. (a) What is the width of a single slit that produces its first minimum at \(\displaystyle 60.0°\) for 600-nm light? (b) At what angle will the second minimum be?Ģ0. (a) How wide is a single slit that produces its first minimum for 633-nm light at an angle of \(\displaystyle 28.0°\)? (b) Where is the first minimum for 700-nm red light?ġ9.
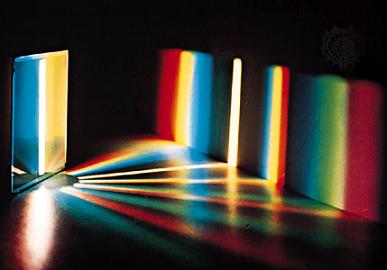
(a) Calculate the angle at which a \(\displaystyle 2.00-μm\)-wide slit produces its first minimum for 410-nm violet light. (a) At what angle is the first minimum for 550-nm light falling on a single slit of width \(\displaystyle 1.00μm\)?ġ8.


 0 kommentar(er)
0 kommentar(er)
